衍生体心立方点阵材料的优化设计及压缩力学行为

许童; 罗嘉琪; 王幸福; 李先雨; 汪聃; 郝刚领; 王新福

doi:10.11973/jxgccl230222

衍生体心立方点阵材料的优化设计及压缩力学行为

1.
延安大学物理与电子信息学院,延安　716000
2.
中国科学院合肥物质科学研究院固体物理研究所,合肥　230000

基金项目:

国家自然科学基金资助项目 12062025

延安大学博士科研启动项目 YDBK2018-20

详细信息

作者简介:
许童（1997—）,男,四川绵阳人,硕士研究生

通讯作者:
王幸福: 王新福副教授

中图分类号: TB303
计量
- 文章访问数: 31
- HTML全文浏览量: 3
- PDF下载量: 8
出版历程
- 收稿日期: 2023-05-11
- 修回日期: 2024-05-11
- 刊出日期: 2024-06-19

Optimization Design and Compressive Mechanical Behavior of Derivative Body-Centered Cubic Lattice Materials

1.
School of Physics and Electronic Information, Yan´an University, Yan´an 716000, China
2.
Institute of Solid State Physics, Chinese Academy of Sciences, Hefei 230000, China

摘要

摘要:
分别在传统体心立方（BCC）点阵结构（H0型）的外围以及外围和中心添加竖直杆,设计出H1型和H2型衍生BCC点阵结构,以AlSi10Mg合金粉末为原料采用激光选区熔化（SLM）技术制备出不同尺寸参数的点阵试样,研究了其压缩力学行为。结果表明：传统和衍生BCC点阵试样的压缩行为均具有线弹性阶段、平台阶段、致密化阶段等3个阶段,属拉伸主导型多孔材料；相同尺寸参数下,H2型BCC点阵试样的比强度、平台应力和单位质量吸能最大,H1型次之,H0型最小。随着单胞高度增加,H1型点阵试样的比强度和单位质量吸能基本不变,平台应力起伏略微增大,能量吸收效率小幅减小；随着单胞宽度增加,H1型点阵试样的比强度、平台应力和单位质量吸能明显降低,能量吸收效率不变；随着斜杆及外围竖直杆杆径增加,H1型点阵试样的比强度、平台应力和单位质量吸能明显增大,能量吸收效率先明显提升后趋于稳定。随着中心竖直杆杆径增加,H2型点阵试样的比强度、平台应力和单位质量吸能增大,能量吸收效率提升。衍生点阵试样的变形模式主要为由下到上的逐层变形,损伤机制为竖直杆的侧向弯曲和斜杆的垂直方向弯曲。
- 衍生体心立方结构 /
- 点阵材料 /
- 优化设计 /
- 压缩力学行为 /
- 吸能特征
Abstract:
By adding vertical rods at periphery and at periphery and center of the traditional body-centered cubic (BCC) lattice structure（H0 type）, the H1 and H2 type derivative BCC lattice structures were designed. The lattice samples with different size parameters were prepared by laser selective melting (SLM) with AlSi10Mg alloy powder as raw materials. The compressive mechanical behaviors of samples were studied. The results show that the traditional and derivative BCC lattice samples were all stretching-oriented porous materials, whose compression behaviors could be divided into three stages: linear elastic stage, plateau stage and densification stage. With the same structural parameters, the specific strength, platform stress and energy absorption per unit mass of H2 type BCC lattice sample were the largest, followed by those of H1 type, and those of H0 type were the smallest. With the increase of cell height, the specific strength and energy absorption per unit mass of H1 type lattice samples were basically unchanged, the fluctuation of the platform stress increased slightly, and the energy absorption efficiency decreased slightly. With the increase of cell width, the specific strength, platform stress and energy absorption per unit mass of H1 type lattice samples decreased obviously, and the energy absorption efficiency remained unchanged. With the increase of diameter of inclined and peripheral vertical rod, the specific strength, platform stress and energy absorption per unit mass of H1 type lattice samples increased obviously, and the energy absorption efficiency increased first and then became stable. With the increase of diameter of central vertical bar, the specific strength, platform stress, energy absorption per unit mass and energy absorption efficiency of the H2 type lattice samples increased. The deformation mode of the derivative lattice sample was mainly layer by layer deformation from bottom to top, and the damage mechanism was lateral bending of the vertical rod and vertical bending of the inclined rod.
- derivative body-centered cubic structure /
- lattice material /
- optimization design /
- compressive mechanical behavior /
- energy absorption characteristic

HTML全文

0. 引言

点阵材料^[1]是由一种或多种结构单元通过特定方式在空间内排列组合（周期性桁架结构）而形成的具有特殊物理和力学性能的有序多孔材料,因具有较高的孔隙率、良好的能量吸收能力和抗冲击能力,在航空航天、交通、医疗等领域具有广阔的应用前景^[2-9]。与传统多孔材料相比,这种有序多孔材料具有更强的可设计性和更高的比强度、比刚度和单位质量吸能性,是目前国际上公认的最具发展潜力的轻质超强韧结构材料之一。近年来,增材制造技术的发展也极大推动了点阵材料的发展,使得复杂结构和微小尺寸点阵材料的制备成为可能^[10-13]。

根据结构单元的排列方式,点阵材料可分为二维点阵材料和三维点阵材料。二维点阵材料主要是指由多边形二维排列组成并在第三方向拉伸成棱柱状的蜂窝材料,也称为格栅材料,主要构型包括三角形、正方形、六边形等；三维点阵材料则是由杆、板等微构件按一定规律重复排列而成的具有空间点阵结构的材料^[10-13],主要构型包括四面体、八面体、立方体、三维Kagome等^[14-15]。研究表明,在相同的孔隙率下,三维点阵材料的刚度和承载能力相比二维点阵材料更强,更适用于缓冲吸能和冲击防护^[16-20]。

在众多点阵材料的拓扑结构中,体心立方（BCC）点阵是一种常见的结构,具有结构简单、能量吸收优势明显、可制备性强等特点,因而受到国内外学者的广泛关注。BCC点阵材料的性能可以通过改变BCC点阵结构单胞的尺寸、数量和杆径等参数进行优化。DAR等^[21]研究发现：在相对密度固定的情况下,一定体积内随着BCC点阵结构单胞数量的增加,点阵材料的弹性模量、屈服应力和平台应力均增大,致密化应变减小；压缩试验后小尺寸单胞点阵材料表现出逐层递进式坍塌,而大尺寸单胞点阵材料则表现出屈曲效应,并伴有中心局部变形,说明小尺寸单胞点阵结构更加稳定,材料的抗压强度更高。DONG等^[22]通过激光选区熔化（SLM）技术制备了BCC点阵材料,其弹性模量和屈服强度均随着单胞杆径减小而降低。ZHAO等^[23]提出了使用锥形杆件构建传统BCC点阵结构的建模方法,并通过有限元分析和单轴压缩试验研究了该点阵材料的力学性能,结果表明锥形杆件显著降低了BCC点阵结构的各向异性,并使材料的弹性模量提高了67%。MEYER等^[24]通过对BCC点阵结构的杆件连接处进行加强处理,获得了两种缺口应力优化方法,降低了3D打印缺口对点阵材料力学性能的不利影响。PLOCHER等^[25]研究发现,与均匀密度的BCC点阵材料相比,压缩载荷下具有适度密度梯度的BCC点阵材料的刚度有一定提高,但继续增大密度梯度则会导致其刚度下降。DONG^[26]将简单立方（SC）和BCC点阵结构通过卡扣连接组合,采用真空钎焊技术制备了Ti-6Al-4V合金衍生BCC点阵材料,压缩试验结果表明衍生BCC点阵材料比传统BCC点阵材料具有更高的承载能力。

目前,对BCC点阵结构优化的研究主要集中在BCC点阵结构单胞尺寸、杆径、梯度和缺陷等方面,而对杆件数量、连接方式的研究较少。此外,由于涉及到局部微结构变化,不仅建模难度会增加,3D打印试样的精度也难以保证。基于此,作者提出了一种具有竖直支撑杆件的衍生BCC结构,并对其进行参数化设计,采用SLM技术制备了不同结构及参数的AlSi10Mg合金点阵试样,通过压缩试验测试了试样的力学行为和吸能特性,并对其变形模式及损伤机理进行了分析,以期为轻量化强韧点阵结构的设计提供理论依据,并为实际应用提供参考。

1. 试样制备与试验方法

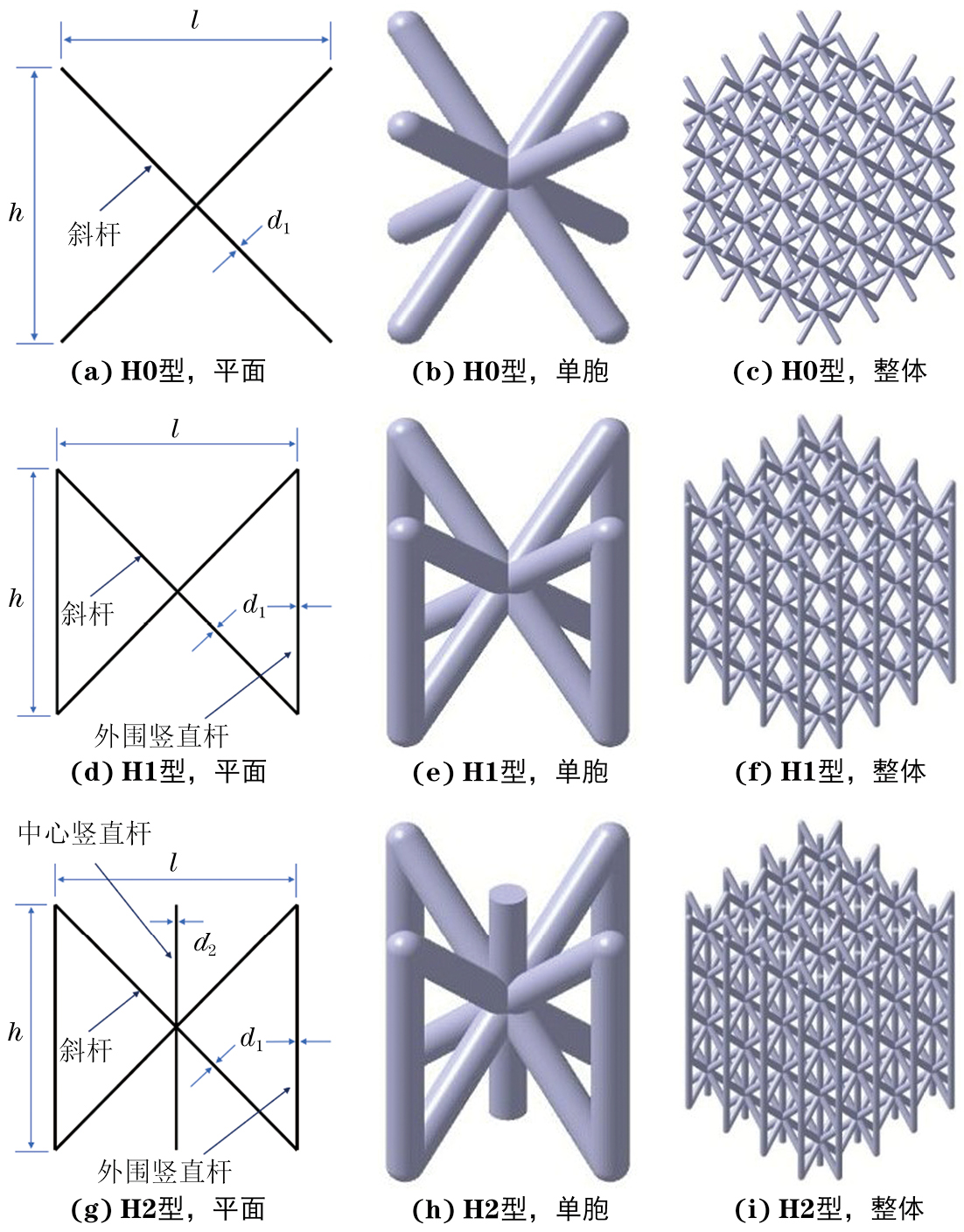
采用Catia软件进行建模设计,分别在传统BCC点阵结构（H0型）的单胞外围以及外围和中心添加竖直杆,设计出H1型和H2型2种衍生BCC点阵结构。3种BCC点阵结构的平面结构、单胞结构及整体结构如图1所示；整体结构由单胞在x,y和z方向进行线性阵列得到。单胞的主要参数包括单胞高度h、单胞宽度l、单胞相对密度 $\bar{ρ}$ 、斜杆/外围竖直杆杆径d₁、中心竖直杆杆径d₂,具体数值见表1。其中, $\bar{ρ}$ 采用公式计算得到,公式如下：

式中：ρ₀,ρ_s分别为单胞的表观密度和实体杆密度。

图 1 3种BCC点阵结构的平面、单胞和整体结构

Figure 1. Planar (a, d, g), single cell (b, e, h) and overall (c, f, i) structures of three kinds of BCC lattice structures:(a‒c) H0 type; (d‒f) H1 type and (g‒i) H2 type

下载: 全尺寸图片幻灯片

表 1 3种BCC点阵结构单胞的主要参数

Table 1. Main parameters of single cell of three kinds of BCC lattice structures

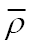
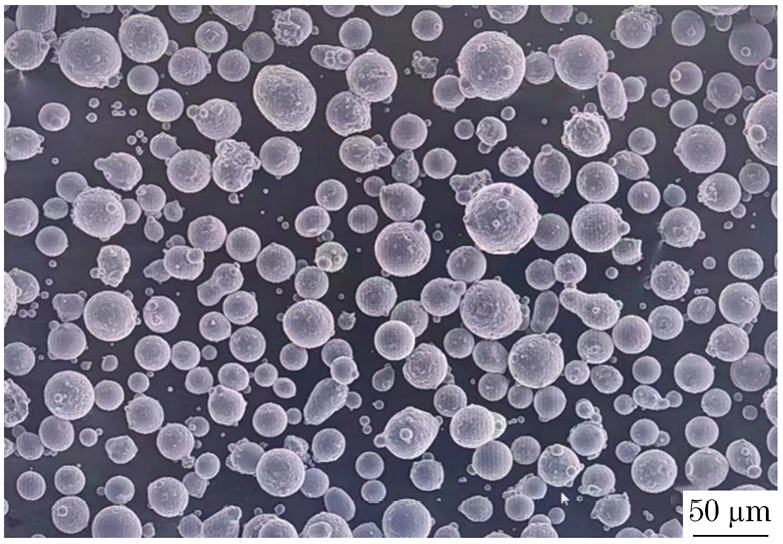
结构类型	试样序号	h/mm	l/mm	d₁/mm	d₂/mm	$\bar{ρ}$
H0	1	6	6	0.8		0.069
H1	2	5	6	0.8		0.101
	3	6	6	0.8		0.093
	4	7	6	0.8		0.087
	5	6	5	0.8		0.125
	6	6	7	0.8		0.073
	7	6	6	0.6		0.052
	8	6	6	1.0		0.145
H2	9	6	6	0.8	0.6	0.100
	10	6	6	0.8	0.8	0.107
	11	6	6	0.8	1.0	0.115

下载: 导出CSV

| 显示表格

按照表1中设计的单胞参数,采用SLM550型三维打印机在x,y和z方向各制备4个单胞而获得BCC点阵试样。试验原料为AlSi10Mg合金粉末,由武汉易成三维科技有限公司提供,粉末颗粒呈球形（见图2）,粒径在15~53 μm,分布较为均匀,化学成分（质量分数/%）为9.0Si,0.55Fe,0.05Cu,0.2~0.45Mn,0.25~0.45Mg,0.05Ni,0.10Zn,0.05Pb,0.05Sn,0.15Ti,余Al。打印时的激光功率为500 W,扫描速度为1 300 mm·s⁻¹,扫描间距为0.1 mm,铺粉层厚为100 μm。打印结束后,为提高点阵试样的强韧性,在SX-GO7123型节能箱式电炉中对其进行280 ℃×2 h的退火处理。

图 2 AlSi10Mg合金粉末的微观形貌

Figure 2. Micromorphology of AlSi10Mg alloy powder

下载: 全尺寸图片幻灯片

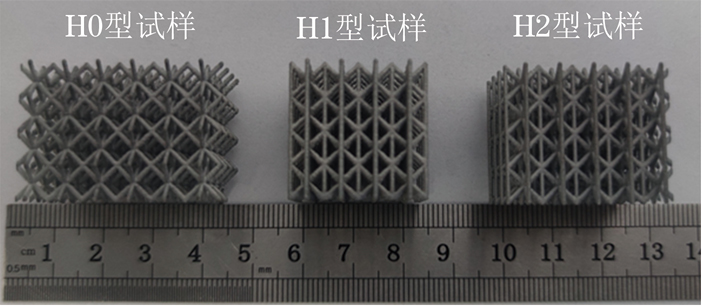
制备的3种点阵结构试样的宏观形貌如图3所示（每种结构举一例）。在试验条件下,不同尺寸参数、不同点阵结构试样的整体成形效果均良好,与三维模型吻合,杆件表面较为光滑,无明显缺陷。采用Model 43 MTS型万能试验机对点阵试样进行准静态压缩试验,应变速率为1.4×10⁻³ s⁻¹。根据获得的压缩应力-应变曲线,计算单位质量吸能、能量吸收效率、比强度和平台应力,公式如下：

W_{s} = \frac{\int_{0}^{ε_{D}} σ (ε) d ε}{\bar{ρ} ρ_{s}}

(3)

η = \frac{\int_{0}^{ε} σ (ε) d ε}{σ_{p}^{*}}

(4)

σ_{ss} = \frac{σ_{s}}{ρ_{0}}

(5)

σ_{pl} = \frac{\int_{0}^{ε_{D}} σ (ε) d ε}{ε_{D}}

(6)

式中：W_s为单位质量吸能；ε_D为致密应变,即能量吸收效率最大时对应的应变；ε为应变；σ（ε）为应力；ρ_s为AlSi10Mg合金密度；η为能量吸收效率； $σ_{p}^{*}$ 为0~ε应变内的最大应力；σ_ss为比强度；σ_pl为平台应力；σ_s为屈服强度。

图 3 H0、H1和H2型BCC点阵试样的宏观形貌

Figure 3. Macromorphology of H0, H1 and H2 type BCClattice samples

下载: 全尺寸图片幻灯片

2. 试验结果与讨论

2.1 压缩行为和吸能特性

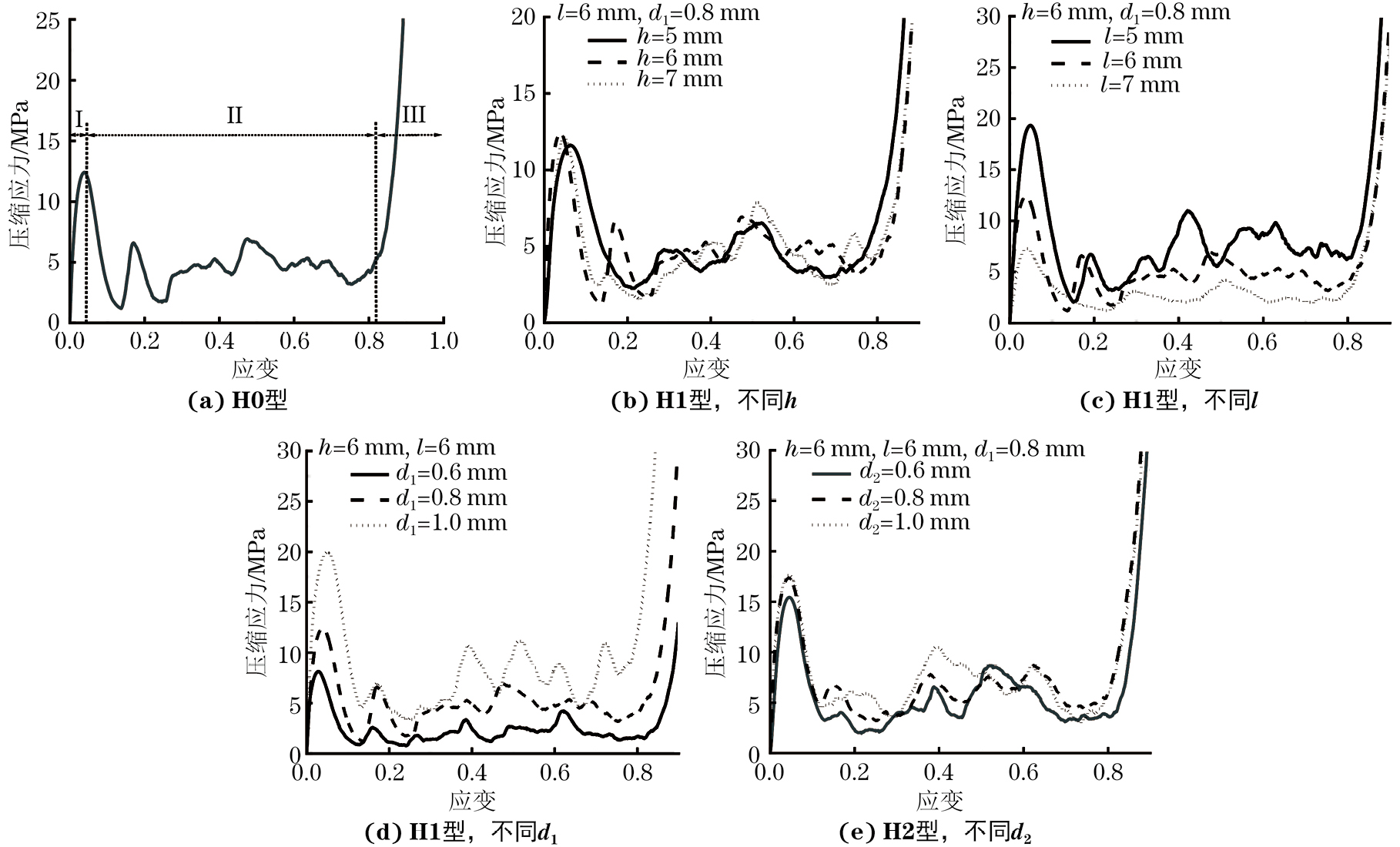
由图4可知：3种BCC点阵试样的压缩过程均可分为3个典型阶段^[27-29],即线弹性阶段（区域I）、平台阶段（区域Ⅱ）和致密化阶段（区域Ⅲ）,应力达到屈服点后明显下降,属拉伸主导型多孔材料。由表2可知：与H0型和H1型BCC点阵试样相比（在相同的单胞高度、单胞宽度、斜杆/外围竖直杆杆径下）,H2型BCC点阵试样的比强度、平台应力和单位质量吸能最大,H1型次之,H0型最小,说明H2型BCC点阵试样的压缩性能和吸能特性最强。

图 4 3种BCC点阵试样的压缩应力-应变曲线

Figure 4. Compressive stress-strain curves of three kinds of BCC lattice samples: (a) H0 type; (b) H1 type with different values of h; (c) H1 type with different values of l; (d) H1 type with different values of d₁ and (e) H2 type with different values of d₂

下载: 全尺寸图片幻灯片

表 2 3种BCC点阵试样的力学性能

Table 2. Mechanical properties of three kinds of BCC lattice samples

结构类型	试样序号	σ_s/MPa	σ_ss/(N·m·g⁻¹)	σ_ss/MPa	ε_D	W_s/(J·g⁻¹)
H0	1	3.14	16.94	0.78	0.88	3.70
H1	2	11.60	41.43	5.07	0.84	15.05
	3	12.41	45.79	4.97	0.86	15.98
	4	12.12	45.22	4.77	0.86	15.87
	5	19.32	55.36	7.81	0.86	19.38
	6	7.29	35.56	2.93	0.84	11.97
	7	8.13	39.85	2.45	0.89	10.48
	8	19.94	57.46	8.29	0.82	19.83
H2	9	15.43	51.78	5.52	0.87	15.91
	10	17.35	55.43	6.79	0.85	18.44
	11	17.64	55.65	7.27	0.85	19.78

下载: 导出CSV

| 显示表格

随着单胞高度增加,H1型点阵试样的比强度和单位质量吸能基本不变,这主要是因为在试验高度范围内竖直杆的抗弯能力基本相同；平台应力起伏略微增大,这是材料相对密度随单胞高度增加而降低导致其稳定性下降所致。随着单胞宽度增加,H1型点阵试样的比强度、平台应力和单位质量吸能明显降低,这一方面是因为试样的相对密度有所降低,另一方面是因为单胞斜杆长度增加且与水平方向的夹角减小使得杆件在加载方向承载分量降低。随着单胞斜杆及外围竖直杆杆径增加,H1型点阵试样的比强度、平台应力和单位质量吸能均明显提升,这是因为杆件的刚度和抗压强度随杆径增加而增大,抗弯能力增强,同时点阵试样相对密度的提高也使其更加稳定；随着单胞中心竖直杆杆径增加,H2型点阵试样的比强度、平台应力和单位质量吸能增大,这同样得益于抗弯能力的增强和相对密度的提高。

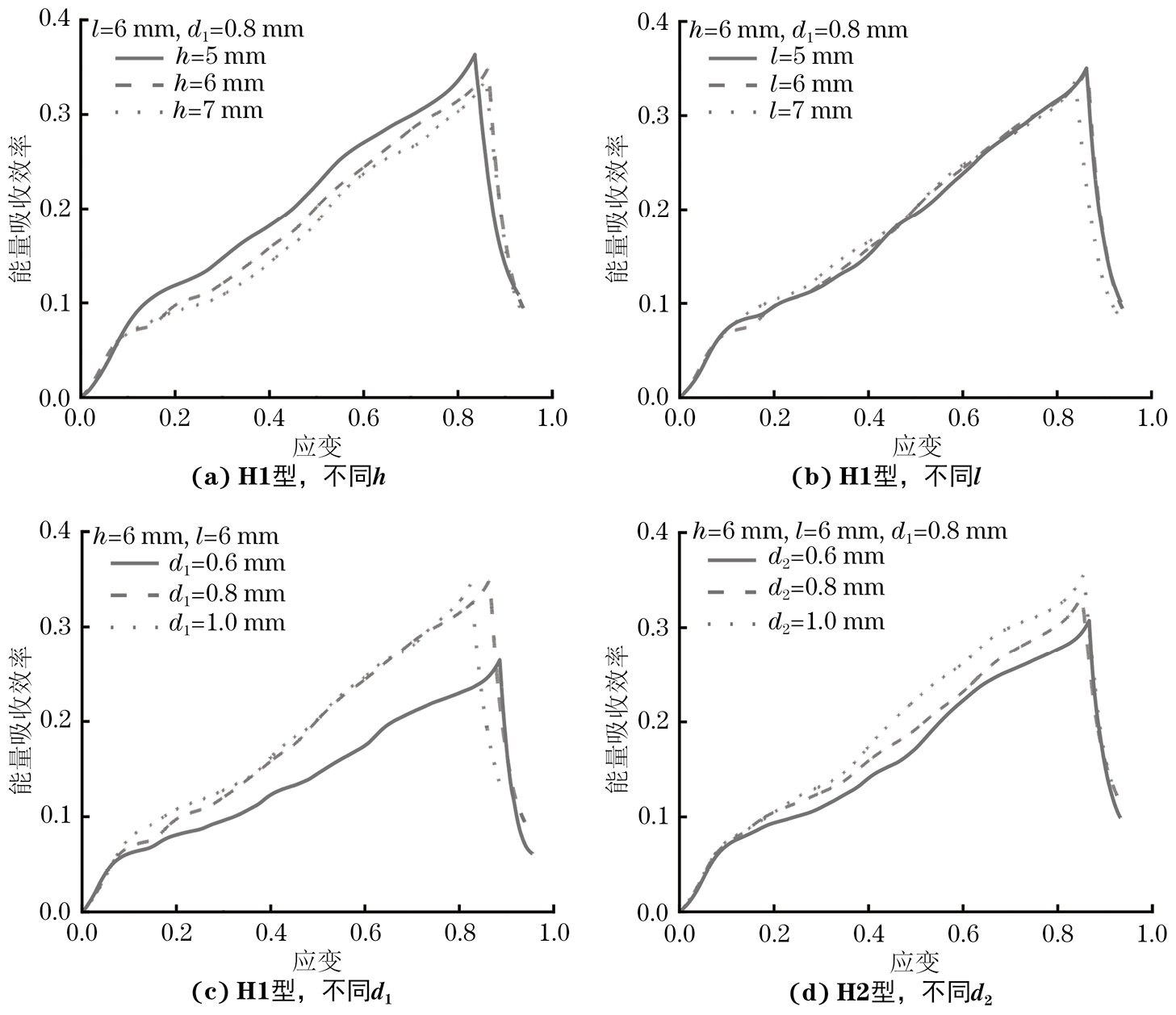
由图5可见：H1、H2型BCC点阵试样在弹性阶段的能量吸收效率随应变增加快速增大,到达屈服点后增大速度变慢,这是由于平台阶段应力降低导致的；达到致密应变附近,能量吸收效率开始快速减小,这是因为致密化阶段峰值应力迅速升高而试样吸能较少。随着单胞高度增加,H1型BCC点阵试样的能量吸收效率减小,但减小幅度不大；随着斜杆和外围竖直杆杆径增加,能量吸收效率显著增大,但当杆径增加至0.8 mm及以上时,能量吸收效率变化不再明显,说明斜杆和外围竖直杆杆径增加对能量吸收效率的提升存在临界效应；单胞宽度对能量吸收效率基本没有影响。随着中心竖直杆杆径增加,H2型BCC点阵试样的能量吸收效率呈现一定幅度的提升。

图 5 H1、H2型BCC点阵试样的能量吸收效率随应变的变化曲线

Figure 5. Variation curves of energy absorption efficiency vs strain of H1 and H2 type BCC lattice samples: (a) H1 type with different h;(b) H1 type with different l; (c) H1 type with different d₁ and (d) H2 type with different d₂

下载: 全尺寸图片幻灯片

2.2 变形模式

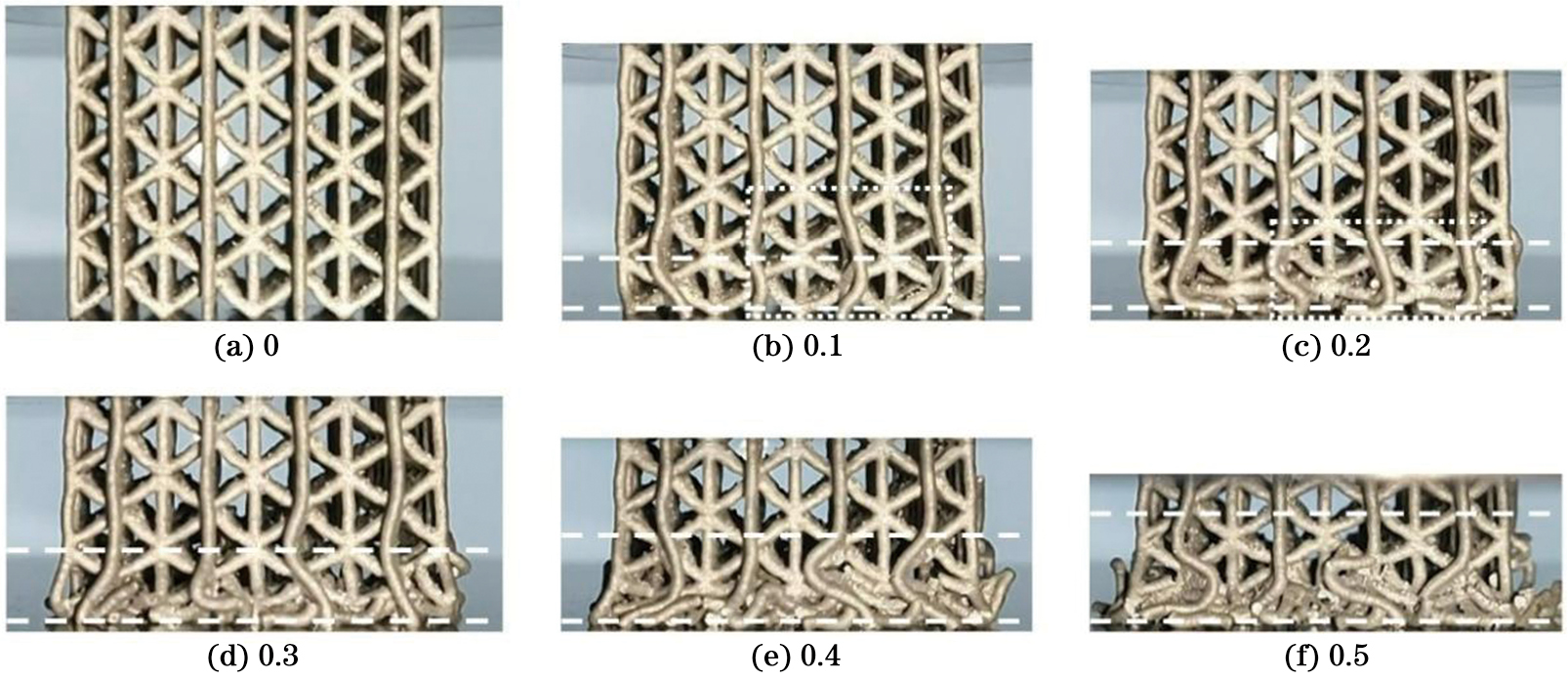
不同结构参数下衍生BCC点阵试样的变形模式大致相似,以h,l,d₁分别为5,6,0.8 mm的H1型BCC点阵试样为例进行分析。由图6可见,在初始阶段,试样最下层先变形,竖直杆在应力作用下向两侧弯曲,斜杆在垂直方向上弯曲变形。当应变为0.1时,变形区域（方框圈出部分）中竖直杆的变形幅度大于斜杆,这是因为竖直杆承受的力大于斜杆,并且斜杆构成的交叉结构比竖直杆稳固。随着应变增加,变形区域向上层传递并逐渐扩大,这是因为在压缩过程中,竖直杆的应变速率大于斜杆,当竖直杆因大幅变形甚至断裂失去支撑作用时,一部分应力便转移到斜杆上,使斜杆承受的应力增加,当斜杆也失效时,应力便传递到邻近区域的单胞层,因此呈现逐层变形趋势。当应变达到0.4~0.5时,部分杆件开始断裂,这是因为压缩过程中杆件随应变增加而变形并向不同方向移动,杆与杆相互拉扯或挤压,导致杆连接处产生较大应力集中,从而发生断裂。

图 6 不同应变下H1型BCC点阵试样的形貌（h=5 mm,l=6 mm,d₁=0.8 mm）

Figure 6. Morphology of H1 type BCC lattice sample at different strains (h=5 mm,l=6 mm,d₁=0.8 mm)

下载: 全尺寸图片幻灯片

3. 结论

（1）传统和衍生BCC点阵试样的压缩过程均包括线弹性阶段、平台阶段、致密化阶段等3个阶段,属拉伸主导型多孔材料。在外围和中心均添加与H0型BCC点阵结构中斜杆杆径相同的竖直杆而得到的H2型BCC点阵试样的比强度、平台应力和单位质量吸能最大,仅在外围添加竖直杆的H1型BCC点阵试样次之,传统的H0型BCC点阵试样最小。

（2）随着单胞高度增加,H1型点阵试样的比强度和单位质量吸能基本不变,平台应力起伏略微增大,能量吸收效率小幅减小；随着单胞宽度增加,比强度、平台应力和单位质量吸能明显降低,能量吸收效率不变；随着单胞斜杆及外围竖直杆杆径增加,比强度、平台应力和单位质量吸能明显增大,能量吸收效率先明显提升后趋于稳定。随着单胞中心竖直杆杆径增加,H2型点阵试样的比强度、平台应力和单位质量吸能增大,能量吸收效率提升。

（3）衍生BCC点阵试样的变形模式主要为由下到上的逐层变形,损伤机制为竖直杆的侧向弯曲和斜杆的垂直方向弯曲。

图 1 3种BCC点阵结构的平面、单胞和整体结构

Figure 1. Planar (a, d, g), single cell (b, e, h) and overall (c, f, i) structures of three kinds of BCC lattice structures:(a‒c) H0 type; (d‒f) H1 type and (g‒i) H2 type

下载: 全尺寸图片幻灯片

图 2 AlSi10Mg合金粉末的微观形貌

Figure 2. Micromorphology of AlSi10Mg alloy powder

下载: 全尺寸图片幻灯片

图 3 H0、H1和H2型BCC点阵试样的宏观形貌

Figure 3. Macromorphology of H0, H1 and H2 type BCClattice samples

下载: 全尺寸图片幻灯片

图 4 3种BCC点阵试样的压缩应力-应变曲线

下载: 全尺寸图片幻灯片

图 5 H1、H2型BCC点阵试样的能量吸收效率随应变的变化曲线

下载: 全尺寸图片幻灯片

图 6 不同应变下H1型BCC点阵试样的形貌（h=5 mm,l=6 mm,d₁=0.8 mm）

Figure 6. Morphology of H1 type BCC lattice sample at different strains (h=5 mm,l=6 mm,d₁=0.8 mm)

下载: 全尺寸图片幻灯片

表 1 3种BCC点阵结构单胞的主要参数

Table 1 Main parameters of single cell of three kinds of BCC lattice structures

结构类型	试样序号	h/mm	l/mm	d₁/mm	d₂/mm	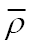$\bar{ρ}$
H0	1	6	6	0.8		0.069
H1	2	5	6	0.8		0.101
	3	6	6	0.8		0.093
	4	7	6	0.8		0.087
	5	6	5	0.8		0.125
	6	6	7	0.8		0.073
	7	6	6	0.6		0.052
	8	6	6	1.0		0.145
H2	9	6	6	0.8	0.6	0.100
	10	6	6	0.8	0.8	0.107
	11	6	6	0.8	1.0	0.115

下载: 导出CSV

表 2 3种BCC点阵试样的力学性能

Table 2 Mechanical properties of three kinds of BCC lattice samples

结构类型	试样序号	σ_s/MPa	σ_ss/(N·m·g⁻¹)	σ_ss/MPa	ε_D	W_s/(J·g⁻¹)
H0	1	3.14	16.94	0.78	0.88	3.70
H1	2	11.60	41.43	5.07	0.84	15.05
	3	12.41	45.79	4.97	0.86	15.98
	4	12.12	45.22	4.77	0.86	15.87
	5	19.32	55.36	7.81	0.86	19.38
	6	7.29	35.56	2.93	0.84	11.97
	7	8.13	39.85	2.45	0.89	10.48
	8	19.94	57.46	8.29	0.82	19.83
H2	9	15.43	51.78	5.52	0.87	15.91
	10	17.35	55.43	6.79	0.85	18.44
	11	17.64	55.65	7.27	0.85	19.78

下载: 导出CSV

参考文献(29)

[1]	EVANS A G,HUTCHINSON J W,FLECK N A,et al. The topological design of multifunctional cellular metals[J]. Progress in Materials Science,2001,46（3/4）：309-327.
[2]	SORO N ,ATTAR H ,BRODIE E ,et al. Evaluation of the mechanical compatibility of additively manufactured porous Ti-25Ta alloy for load-bearing implant applications[J]. Journal of the Mechanical Behavior of Biomedical Materials,2019,97：149-158.
[3]	DUMAS M ,TERRIAULT P ,BRAILOVSKI V. Modelling and characterization of a porosity graded lattice structure for additively manufactured biomaterials[J]. Materials & Design,2017,121：383-392.
[4]	王向明,苏亚东,吴斌,等. 微桁架点阵结构在飞机结构/功能一体化中的应用[J]. 航空制造技术,2018,61（10）：16-25. WANG X M ,SU Y D ,WU B ,et al. Application for additive manufacturing of lattice materials on integrated aircraft structures and functions[J]. Aeronautical Manufacturing Technology,2018,61（10）：16-25.
[5]	杨鑫,马文君,王岩,等. 增材制造金属点阵多孔材料研究进展[J]. 材料导报,2021,35（7）：7114-7120. YANG X ,MA W J ,WANG Y ,et al. Research progress of metal lattice porous materials for additive manufacturing[J]. Materials Reports,2021,35（7）：7114-7120.
[6]	GEBHARDT U ,GUSTMANN T ,GIEBELER L ,et al. Additively manufactured AlSi10Mg lattices：Potential and limits of modelling as-designed structures[J]. Materials & Design,2022,220：110796.
[7]	YU X L ,ZHOU J ,LIANG H Y ,et al. Mechanical metamaterials associated with stiffness,rigidity and compressibility：A brief review[J]. Progress in Materials Science,2018,94：114-173.
[8]	SHARMA D ,HIREMATH S S. Bio-inspired repeatable lattice structures for energy absorption：Experimental and finite element study[J]. Composite Structures,2022,283：115102.
[9]	RAHMANI R ,ANTONOV M ,KOLLO L ,et al. Mechanical behavior of Ti6Al4V scaffolds filled with CaSiO₃ for implant applications[J]. Applied Sciences,2019,9（18）：3844.
[10]	HELOU M ,KARA S. Design,analysis and manufacturing of lattice structures：An overview[J]. International Journal of Computer Integrated Manufacturing,2018,31（3）：243-261.
[11]	许德,高华兵,董涛,等. 增材制造用金属粉末研究进展[J]. 中国有色金属学报,2021,31（2）：245-257. XU D ,GAO H B ,DONG T ,et al. Research progress of metal powder for additive manufacturing[J]. The Chinese Journal of Nonferrous Metals,2021,31（2）：245-257.
[12]	耿鹏,陈道兵,周燕,等. 增材制造智能材料研究现状及展望[J]. 材料工程,2022,50（6）：12-26. GENG P ,CHEN D B ,ZHOU Y ,et al. Research status and prospect of additive manufacturing of intelligent materials[J]. Journal of Materials Engineering,2022,50（6）：12-26.
[13]	廉艳平,王潘丁,高杰,等. 金属增材制造若干关键力学问题研究进展[J]. 力学进展,2021,51（3）：648-701. LIAN Y P ,WANG P D ,GAO J ,et al. Fundamental mechanics problems in metal additive manufacturing：A state-of-art review[J]. Advances in Mechanics,2021,51（3）：648-701.
[14]	陶斯嘉,王小锋,曾婧,等. 点阵材料及其3D打印[J]. 中国有色金属学报,2022,32（2）：416-444. TAO S J ,WANG X F ,ZENG J ,et al. Lattice materials and its fabrication by 3D printing：A review[J]. The Chinese Journal of Nonferrous Metals,2022,32（2）：416-444.
[15]	CHEN L Y ,LIANG S ,LIU Y J ,et al. Additive manufacturing of metallic lattice structures：Unconstrained design,accurate fabrication,fascinated performances,and challenges[J]. Materials Science and Engineering R,2021,146：100648.
[16]	FAN H L ,YANG W ,ZHOU Q. Experimental research of compressive responses of multi-layered woven textile sandwich panels under quasi-static loading[J]. Composites Part B：Engineering,2011,42（5）：1151-1156.
[17]	ZHENG J J ,ZHAO L ,FAN H L. Energy absorption mechanisms of hierarchical woven lattice composites[J]. Composites Part B：Engineering,2012,43（3）：1516-1522.
[18]	LU T J ,ZHANG Q C ,FENG J. Recent progress in the development of lightweight porous materials and structures[J]. Materials China,2012,31：13-25.
[19]	BANHART J. Light-metal foams：History of innovation and technological challenges[J]. Advanced Engineering Materials,2013,15：82-111.
[20]	IVAÑEZ I ,FERNANDEZ-CAÑADAS L M ,SANCHEZ-SAEZ S. Compressive deformation and energy-absorption capability of aluminium honeycomb core[J]. Composite Structures,2017,174：123-133.
[21]	DAR U A ,MIAN H H ,ABID M ,et al. Experimental and numerical investigation of compressive behavior of lattice structures manufactured through projection micro stereolithography[J]. Materials Today Communications,2020,25：101563.
[22]	DONG Z C ,ZHANG X Y ,SHI W H ,et al. Study of size effect on microstructure and mechanical properties of AlSi10Mg samples made by selective laser melting[J]. Materials,2018,11（12）：2463.
[23]	ZHAO M ,LI X W ,ZHANG D ,et al. Design,mechanical properties and optimization of lattice structures with hollow prismatic struts[J]. International Journal of Mechanical Sciences,2023,238：107842.
[24]	MEYER G ,WANG H X ,MITTELSTEDT C. Influence of geometrical notches and form optimization on the mechanical properties of additively manufactured lattice structures[J]. Materials & Design,2022,222：111082.
[25]	PLOCHER J ,PANESAR A. Effect of density and unit cell size grading on the stiffness and energy absorption of short fibre-reinforced functionally graded lattice structures[J]. Additive Manufacturing,2020,33：101171.
[26]	DONG L. Mechanical responses of Ti-6Al-4V truss lattices having a combined simple-cubic and body-centered-cubic（SC-BCC）topology[J]. Aerospace Science and Technology,2021,116：106852.
[27]	WANG P ,YANG F ,LI P H ,et al. Design and additive manufacturing of a modified face-centered cubic lattice with enhanced energy absorption capability[J]. Extreme Mechanics Letters,2021,47：101358.
[28]	LEI H S ,LI C L ,MENG J X ,et al. Evaluation of compressive properties of SLM-fabricated multi-layer lattice structures by experimental test and μ-CT-based finite element analysis[J]. Materials & Design,2019,169：107685.
[29]	DESHPANDE V S ,ASHBY M F ,FLECK N A. Foam topology：Bending versus stretching dominated architectures[J]. Acta Materialia,2001,49（6）：1035-1040.

施引文献

资源附件(0)

图(6) / 表(2)

计量

文章访问数: 31
HTML全文浏览量: 3
PDF下载量: 8
被引次数: 0

0. 引言
1. 试样制备与试验方法
2. 试验结果与讨论
2.1 压缩行为和吸能特性
2.2 变形模式
3. 结论

衍生体心立方点阵材料的优化设计及压缩力学行为

作者简介: 许童（1997—）,男,四川绵阳人,硕士研究生

通讯作者: 王幸福: 王新福副教授

计量

出版历程