Finite Element Simulation of Temperature Field in Swing Arc Narrow Gap GMA Welding Considering Sidewall Heat Source
-
摘要:
基于新型摇动电弧窄间隙熔化极气体保护(GMA)焊的电弧偏转现象,将在侧壁停留时的电弧热源分解为电弧分热源和侧壁热源,建立了考虑侧壁热源的热源模型,采用ANSYS软件建立新型摇动电弧窄间隙GMA焊的有限元模型,对考虑侧壁热源和未考虑侧壁热源时焊接接头横截面熔合线轮廓以及热循环曲线进行模拟,并进行试验验证;采用有限元模拟方法研究了考虑侧壁热源时摇动电弧窄间隙GMA焊接过程中的温度场,并与未考虑侧壁热源时的温度场进行对比。结果表明:模拟得到考虑侧壁热源时焊缝横截面左右两侧壁熔合线最高点的深度与试验结果间的差值小于未考虑侧壁热源时;考虑与不考虑侧壁热源2种条件下,焊接接头不同测试点的热循环模拟曲线的变化趋势与试验结果基本吻合,峰值温度的最大相对误差分别为1.8%和3.4%。考虑侧壁热源的热源模型能更加准确地描述摇动电弧窄间隙GMA焊过程中的热源分布特征。考虑侧壁热源模拟得到的底部熔深略小于未考虑侧壁热源时,而侧壁熔深则略大,但尺寸变化均小于0.1 mm;温度场稳定前,考虑侧壁热源时熔池的形成规律与不考虑侧壁热源的基本一致,但接头上表面的熔池尺寸略小,而温度场稳定后,接头上表面的熔池尺寸也基本一致。
Abstract:Based on the arc deflection of the new type swing arc narrow gap melting electrode gas shield (GMA) welding, the arc heat source staying at the sidewall was decomposed into arc component heat source and sidewall heat source, and the heat source model considering the sidewall heat source was established. The finite element model of the new type swing arc narrow gap GMA welding was established by ANSYS software. The cross-section fusion line profile and thermal cycle curve of welded joint with and without considering sidewall heat source were simulated and verified by experiments. The temperature field in the swing arc narrow gap GMA welding with considering sidewall heat source was studied by the finite element simulation method, and was compared with that without considering sidewall heat source. The results show that the difference between the depth of the highest point of the fusion line on the left and right sides of the weld cross section by simulation with considering sidewall heat source and the test result was smaller than that without considering sidewall heat source. The variation trend of the thermal cycle curves at different test points of the welded joint by simulation with and without considering sidewall heat source was basically consistent with the test results, and the maximum relative errors of peak temperatures were 1.8% and 3.4%, respectively. The heat source model considering the sidewall heat source could more accurately describe the heat source distribution characteristics in the swing arc narrow gap GMA welding. The bottom weld penetration depth by simulation with considering sidewall heat source was smaller than that without considering the sidewall heat source, and the side weld penetration depth was larger, but the size change was less than 0.1 mm. The formation law of the molten pool before the temperature field stabilization was the same as that without considering the sidewall heat source, but the molten pool size on the upper surface of the joint was smaller; after the temperature field was stabilized, the molten pool size on the upper surface of the joint was the same.
-
0. 引言
窄间隙焊接技术选用小角度的坡口,相对大角度坡口断面面积减少50%以上,减少了焊接填充材料的消耗,从而降低了焊接成本[1-2]。目前,常用的窄间隙焊接技术为窄间隙熔化极气体保护(GMA)焊,包括旋转电弧窄间隙GMA焊、双丝窄间隙GMA焊、摇动电弧窄间隙GMA焊,其中新型摇动电弧窄间隙GMA焊通过空心轴步进电机驱动微弯型导电杆绕焊炬中心线往返转动,带动焊丝端部的电弧在坡口内作圆弧形摇动,以实现摇动电弧窄间隙焊接,这种结构的焊炬结构更加紧凑、稳定[3-5]。
焊接温度场对接头性能具有重要影响,相对于薄板的焊接,厚板的温度场更为复杂,对温度场进行研究,对提高焊接接头力学性能非常重要。随着计算机和数值分析技术的发展,数值模拟已成为研究物理过程强有力的工具[6-8]。ELMESALAMY[9]等采用有限元建模研究热循环对激光窄间隙焊接焊缝残余应力的影响。张华军等[10]建立了摆动电弧热源模型,并对摆动式非熔化极惰性气体钨极保护(TIG)平板堆焊温度场进行了模拟计算和分析。
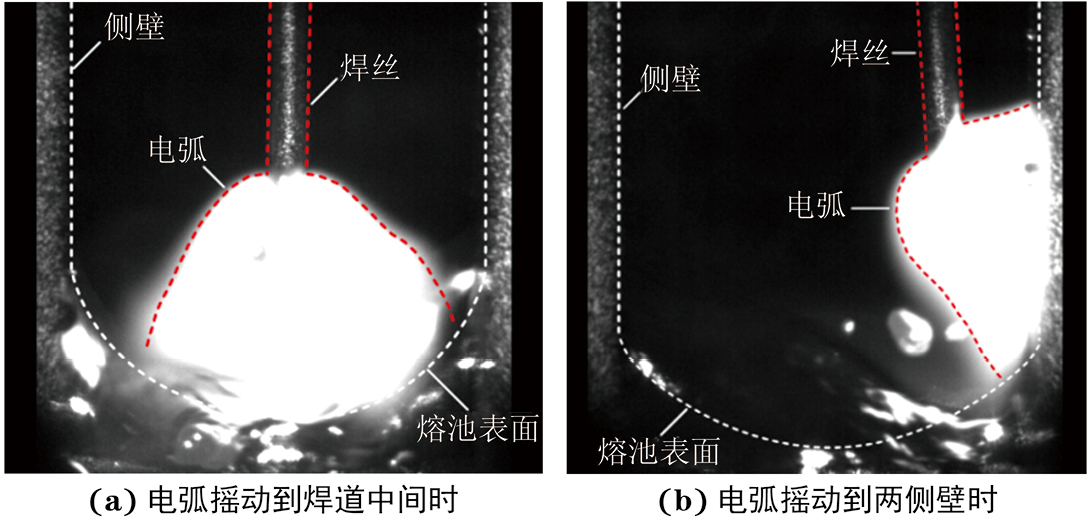
新型摇动电弧窄间隙GMA焊的焊道较窄,当电弧摇动到左右两侧壁时,基于电压最小原理,电弧并不是指向焊丝轴线方向,而是出现了一定程度的偏转,接近垂直于两侧壁。电弧偏转增加了两侧壁对电弧热的吸收,有利于增加侧壁熔深,但是也改变了原有的焊接温度场,使得温度场更加复杂。胥国祥等[11]建立了摇动电弧窄间隙GMA焊温度场数值分析模型,但是未考虑电弧偏转对计算结果的影响。作者在胥国祥等[11]研究的基础上,基于电弧偏转现象建立了考虑侧壁热源的热源模型,采用ANSYS软件建立新型摇动电弧窄间隙GMA焊的有限元模型,对考虑侧壁热源和未考虑侧壁热源时焊接接头横截面熔合线轮廓以及热循环曲线进行模拟,并与试验结果进行对比,同时采用该模拟方法对比研究了考虑侧壁热源和未考虑侧壁热源时焊接接头的温度场,以期为深入了解添加辅丝的摇动电弧窄间隙焊内部机理和焊接工艺参数优化等提供理论基础。
1. 试验方法
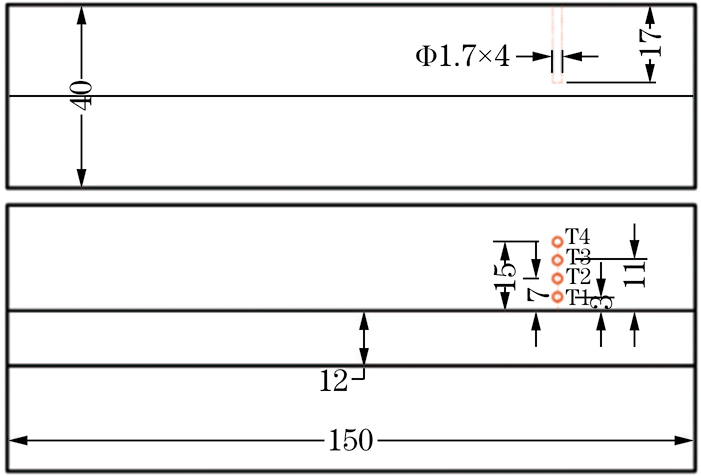
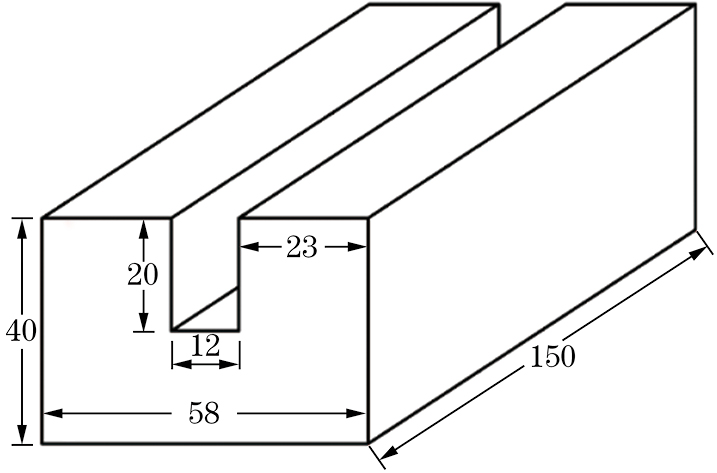
母材为江苏沙钢集团有限公司提供的正火态Q370qE钢;焊丝牌号为ER50-6,直径为1.2 mm。焊接试样尺寸如图1所示。
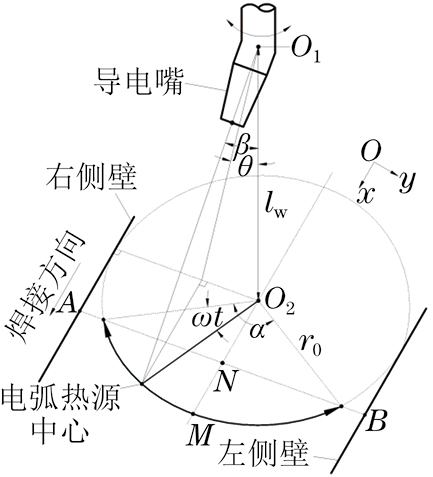
试验用新型摇动电弧窄间隙GMA焊系统由福尼斯TPS 4000型焊接电源、送丝机、行走机构、控制器、焊炬等组成,焊接原理和焊接轨迹如图2所示。在焊接过程中,微弯导电杆绕其中心旋转轴旋转,驱动焊丝端部在一定角度范围内摇动,同时焊炬始终以给定的速度v0沿着焊接方向移动,因此电弧运动由旋转运动和直线运动耦合而成。焊丝从焊道右侧R1点开始起弧,在右侧停留一段时间,此时焊炬沿着x方向运动,因此形成了R1→R2的直线轨迹;焊丝朝着左侧壁摇动,同时焊炬以速度v0沿着焊接方向移动,形成了R2→L1的曲线轨迹;摇动到左侧后,焊丝停留相同的时间,形成L1→L2的直线轨迹;而后从左侧摇动到右侧,形成L2→R3的曲线轨迹。如此反复,完成焊接过程。由图3可见,当电弧摇动到焊道中间时,电弧为正常形态,未发生偏转,而当电弧摇动到焊道两侧壁时,电弧为偏转形态。
新型摇动电弧窄间隙GMA焊接过程中的焊接电流为300 A,电弧电压为28 V,焊接速度为240 mm·min−1,焊丝摇动频率为4 Hz,摇动角度为66°,左右两侧壁停留时间为60 ms,微弯导电杆弯曲角为8°,保护气体为体积分数80%Ar+20%CO2的混合气体。采用K型铠装热电偶测试温度,测温孔的直径为1.7 mm,深度为4 mm,具体位置如图4所示,共测T1,T2,T3,T4等4个测温点。
2. 有限元模型的建立
2.1 传热控制方程
传热控制方程[12]如下:
(1) 式中:H为熔滴热焓;ρ为焊件的密度;κ为焊件的热导率;t为时间;u,v,w分别为x,y,z方向上的焊接速度分量;Sv为内热源强度;T为温度。
2.2 热源模型
在新型摇动电弧窄间隙GMA焊接过程中,当电弧摇动到两侧壁时,电弧同时加热焊缝和侧壁,具有体积热源特点,故采用双椭球体热源描述电弧热输入。然而,当电弧摇动到两侧壁时,电弧几乎垂直于侧壁,使得侧壁吸收的热量增加,进而增加了侧壁熔深,改变了温度场分布,因此为了更加准确地描述热输入,将在两侧壁停留时的热源分解为侧壁热源和电弧分热源,在摇动过程中再将侧壁热源和电弧分热源合并为一个电弧热源。
2.2.1 电弧摇动过程中的热源模型
图5中ω为摇动角速度,ωt为某一时刻电弧摇动角度,α为摇动角度,r0为摇动半径,β为导电杆弯曲角,lw为导电杆弯曲点到工件表面的距离。焊接时,电弧绕着焊炬轴线左右摇动,在垂直焊接方向平面内电弧与焊件侧壁表面呈一定倾斜角度θ,即电弧在垂直焊接方向的平面内存在左右摇动。当电弧摇动到中间时,θ为0°。当电弧从中间摇动到侧壁过程时,θ不断变大,在侧壁停留时达到最大。在这个过程中,电弧热源呈不断变化的倾斜状态。该倾斜角度的存在会影响电弧热流分布模式,从而影响热场特征。同时,由于焊缝表面存在一定的下凹,焊接时电弧热源也并非在平面内左右摇动。综上,必须考虑电弧摇动、焊缝表面形状对温度场的影响。
在电弧摇动过程中采用双椭球体热源描述电弧热输入[11],其热源中心(x1c,y1c,z1c)描述如下:
(2) (3) (4) 式中:z0为热源模型z方向的坐标,由焊缝上表面高度决定。
通过坐标变换的方法推导出电弧热源模型摇动后的热流分布函数:在垂直于焊接方向平面内将热源模型与所在坐标系转动一定的角度,则在新的坐标系(x´,y´,z´)内,热源模型分布函数与原坐标系相同,通过两坐标系之间的坐标变换获得原坐标系下热源模型倾斜后的分布函数。参考文献[11]推导获得原坐标系下具有上述热源中心移动轨迹及倾斜角度的热源模型分布函数,如下:
(5) (6) 式中:qf1为前半部分椭球热流分布函数;qr1为后半部分椭球热流分布函数;η为电弧热效率;I为焊接电流;U为电弧电压;af1,ar1,b1,c1均为热源分布参数。
2.2.2 侧壁停留过程中的热源模型
电弧分热源的热源中心也可由式(2)~式(4)描述,通过坐标转换后得到原坐标系下的热源模型分布函数,如下:
(7) (8) 式中:η1为电弧分热源的热效率。
侧壁热源的中心(x2c,y2c,z2c)[11]可表示如下:
(9) (10) (11) 式中:p为焊件坡口间隙;d为焊趾到焊件底部的距离;b2为热源分布参数。
侧壁热源采用双椭圆平面热源模型[13]描述,表达式为
(12) (13) 式中:qf2为前半部分椭圆热流分布函数;qr2为后半部分椭圆热流分布函数;η2为侧壁热源的热效率;af2,ar2为热源分布参数。
2.3 有限元模型
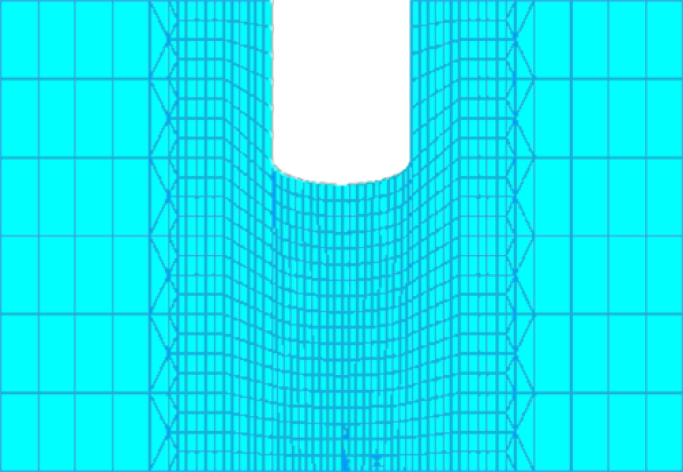
依据摇动电弧窄间隙焊接坡口形式,利用ANSYS有限元软件建立焊接接头的几何模型,并进行网格划分,焊缝区网格尺寸为0.6~1.0 mm,热影响区网格尺寸为2~3 mm,母材区网格尺寸为5 mm左右,采用SOLID70单元,六面体单元网格,具体的网格划分如图6所示。采用生死单元法反映填充金属对焊接传热过程的影响。将上述模型代入传热控制方程,通过ANSYS软件对瞬态温度场进行计算。焊件表面考虑对流及辐射散热,环境及初始温度设为20 ℃,熔点取1 500 ℃。Q370qE钢的热物理性能参数见表1。
表 1 Q370qE钢的热物理性能参数Table 1. Thermophysical performance parameters of Q370qE steel温度/℃ 密度/(g·cm−3) 热导率/(W·m−1·℃−1) 比热容/(J·kg−1·℃−1) 20 7 800 51.9 450 100 7 740 51.1 499 300 7 700 46.1 565 550 7 610 37.5 705 750 7 550 30.6 1 080 1 000 7 490 29.4 437 1 200 7 400 29.7 400 3. 模拟结果与讨论
3.1 试验验证
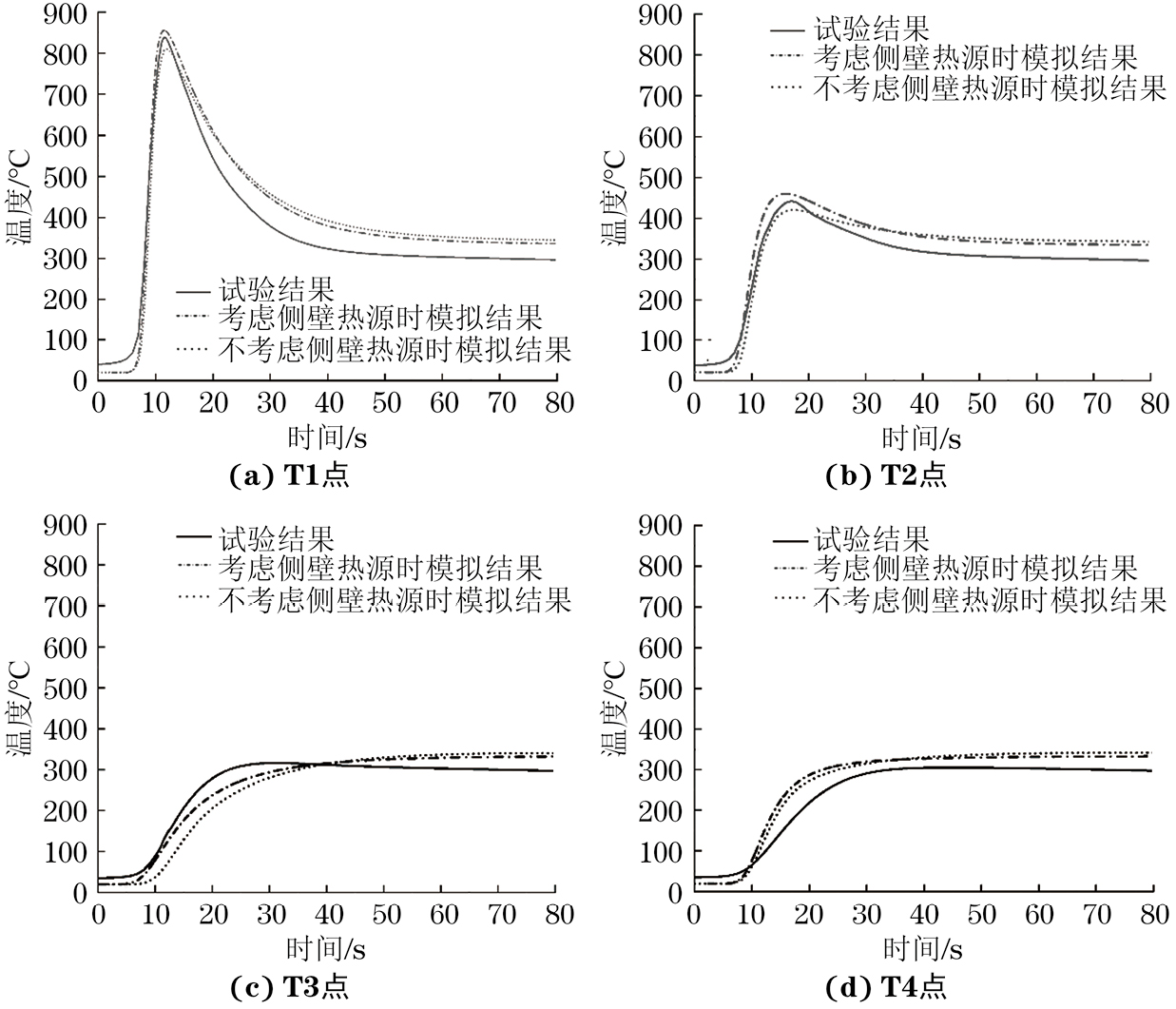
由图7可见:未考虑侧壁热源时,焊缝截面左右两侧壁熔合线最高点的深度相比于试验结果更大,二者相差0.7 mm;考虑侧壁热源时,左右两侧壁熔合线最高点的深度减小,与试验结果相差0.1 mm。由图8可知,考虑与不考虑侧壁热源2种条件下,模拟得到焊接接头不同测试点的热循环曲线的变化趋势与试验结果基本吻合,最大偏差出现在T1点,峰值温度的相对误差分别为1.8%和3.4%。不考虑侧壁热源时,模拟得到的T1点的峰值温度较试验结果低约28 ℃,这是因为不考虑侧壁热源时热源沿着焊丝方向指向熔池底部,热量主要集中在熔池底部,而测温点高度与焊缝上表面相当,因此温度偏低。考虑侧壁热源时将一部分电弧热源分解到侧壁,因此模拟得到的T1点的峰值温度较试验结果高约15 ℃。对比可知,考虑侧壁热源的热源模型能更加准确地描述热源分布特征。
3.2 温度场模拟结果
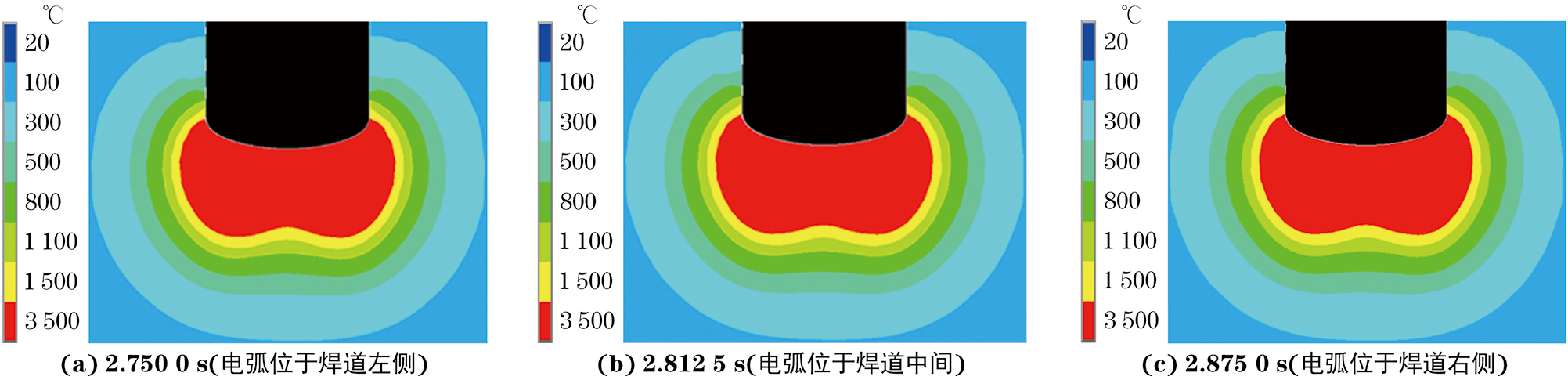
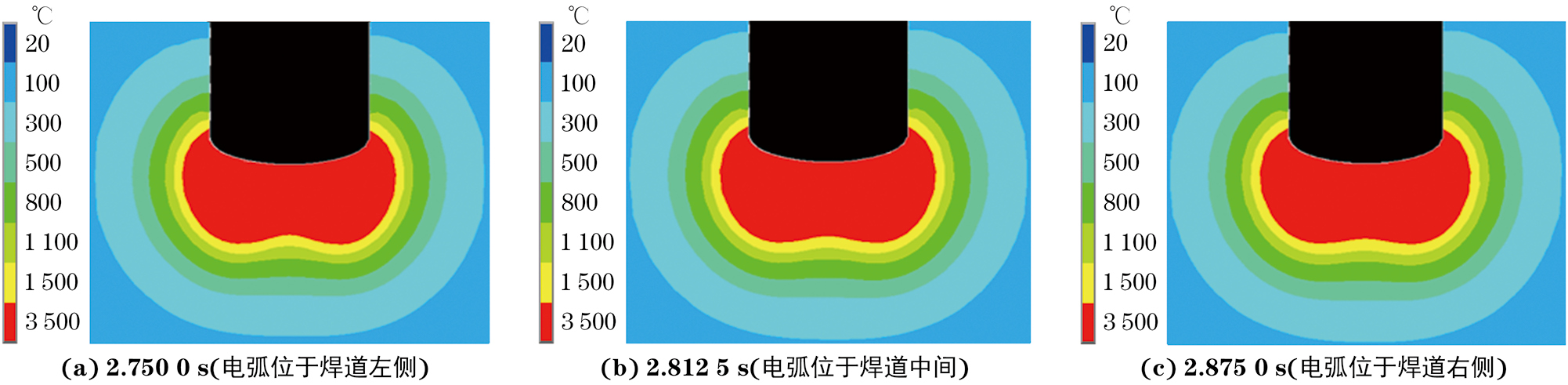
由图9和图10可以看出,在一个周期内,考虑与不考虑侧壁热源2种条件下,焊接接头横截面温度场基本保持稳定,熔池形态基本相同。这是因为电弧热作用具有延迟性,同时电弧摇动频率达到4 Hz,摇动速度很快,还未完全散热焊丝就又在下个周期摇动到该位置,使得横截面温度场基本保持稳定。另外,考虑侧壁热源时的底部熔深略小于未考虑侧壁热源时,而侧壁熔深则略大于未考虑侧壁热源时。这是因为未考虑侧壁热源时热源指向底部,使得底部热量高,熔池变深;而考虑侧壁热源时一部分热量用于加热侧壁,使得侧壁温度升高,熔深变大。但是,侧壁熔深和底部熔深的尺寸变化非常有限,均小于0.1 mm。综上可知,考虑侧壁热源主要影响的是两侧壁的熔合线最高点位置。
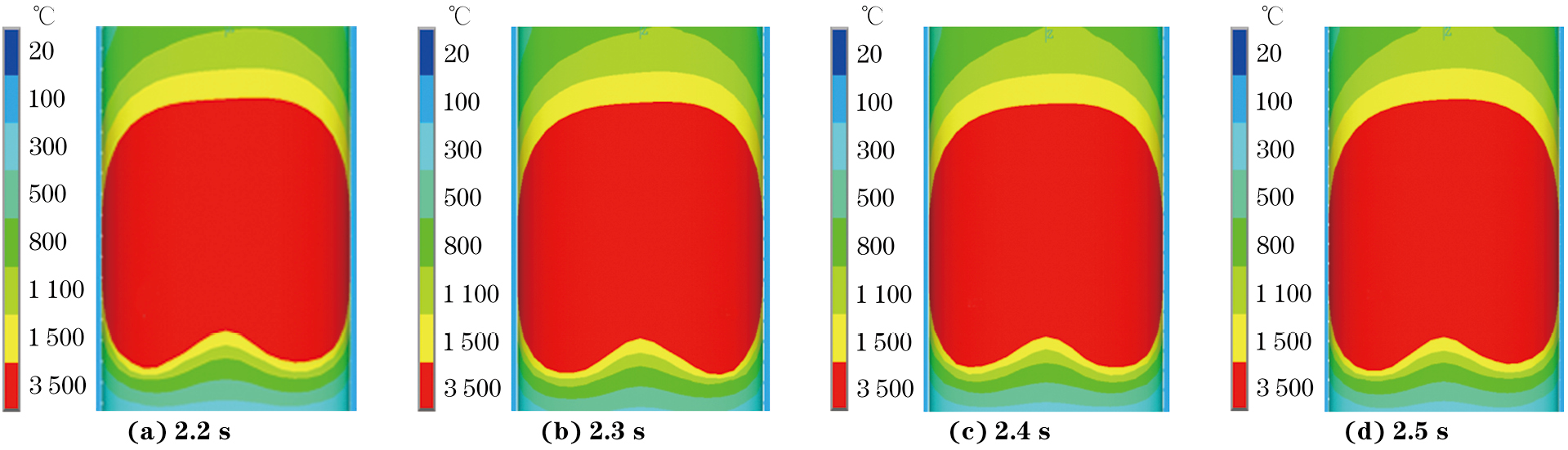
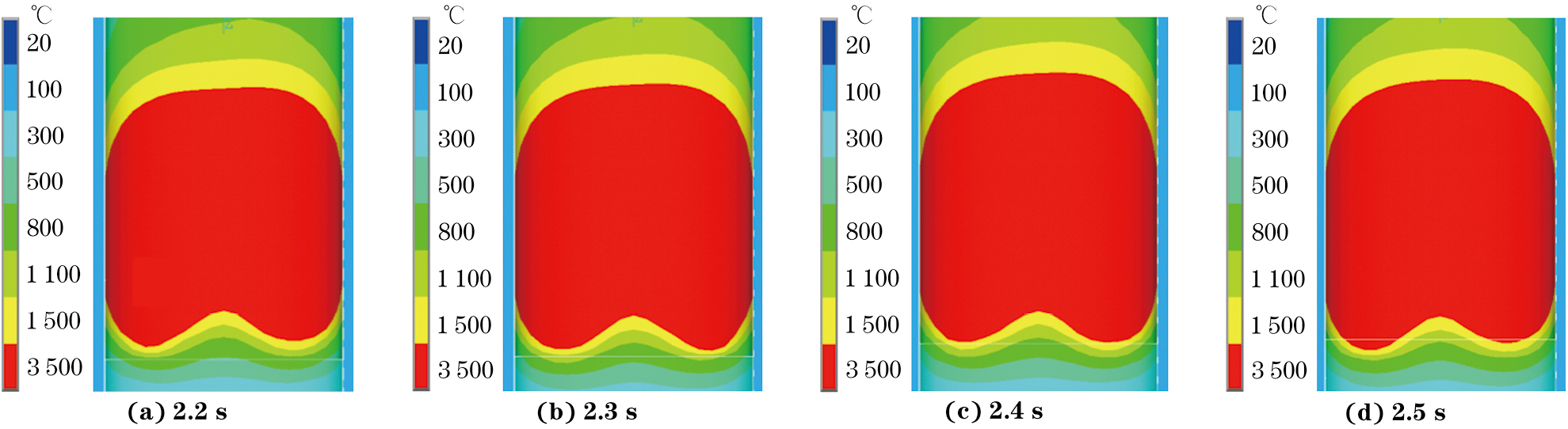
![]() 图 9 未考虑侧壁热源时模拟得到不同时刻焊接接头的横截面温度场Figure 9. Simulated temperature fields on cross-section of welded joint at different times without considering sidewall heat source: (a) 2.750 0 s (arc located on left side of bead); (b) 2.812 5 s (arc located in middle of bead) and (c) 2.875 0 s (arc located on right side of bead)
图 9 未考虑侧壁热源时模拟得到不同时刻焊接接头的横截面温度场Figure 9. Simulated temperature fields on cross-section of welded joint at different times without considering sidewall heat source: (a) 2.750 0 s (arc located on left side of bead); (b) 2.812 5 s (arc located in middle of bead) and (c) 2.875 0 s (arc located on right side of bead)![]() 图 10 考虑侧壁热源时模拟得到不同时刻焊接接头的横截面温度场Figure 10. Simulated temperature fields on cross-section of welded joint at different times with considering sidewall heat source: (a) 2.750 0 s (arc located on left side of bead); (b) 2.812 5 s (arc located in middle of bead) and (c) 2.875 0 s (arc located on right side of bead)
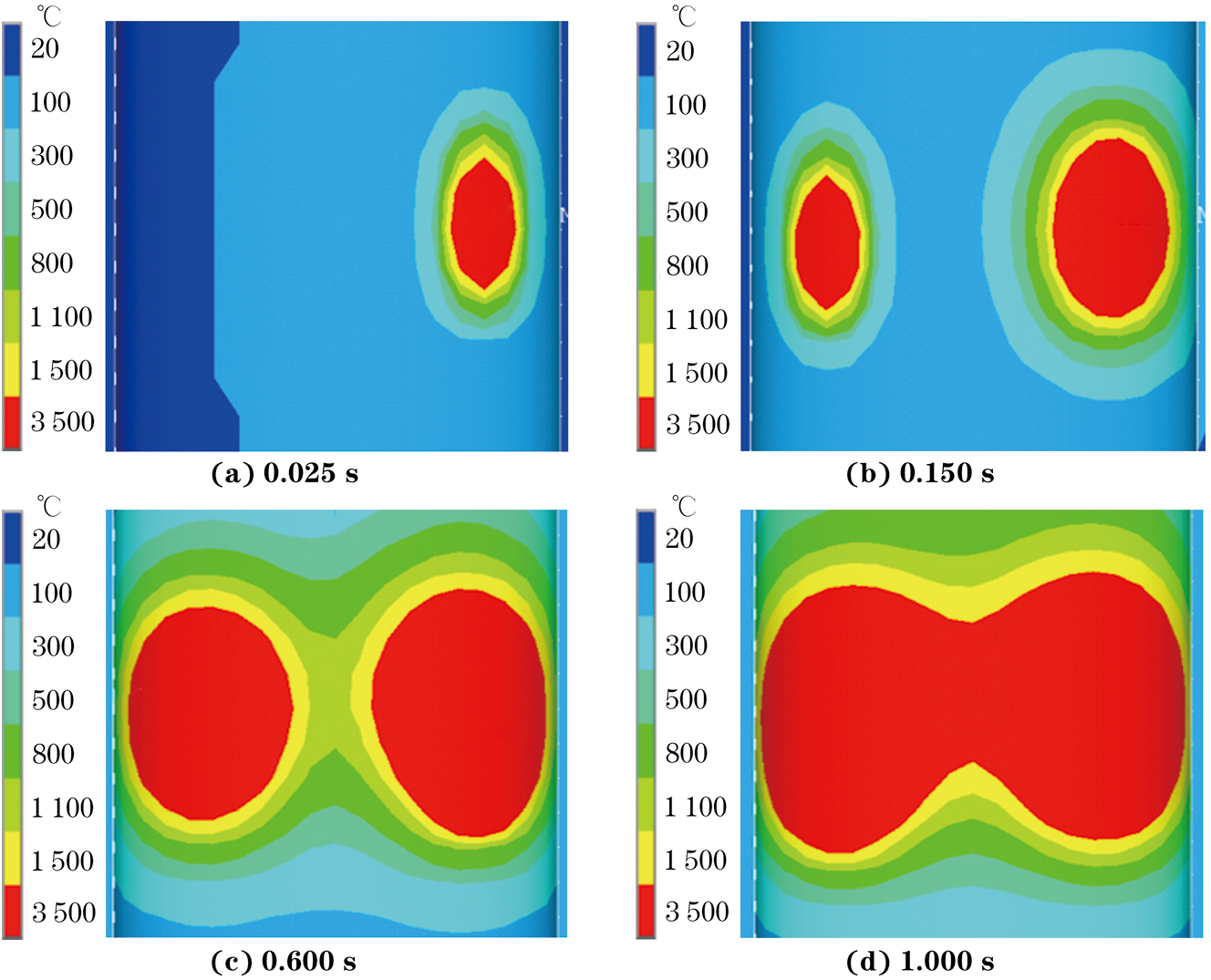
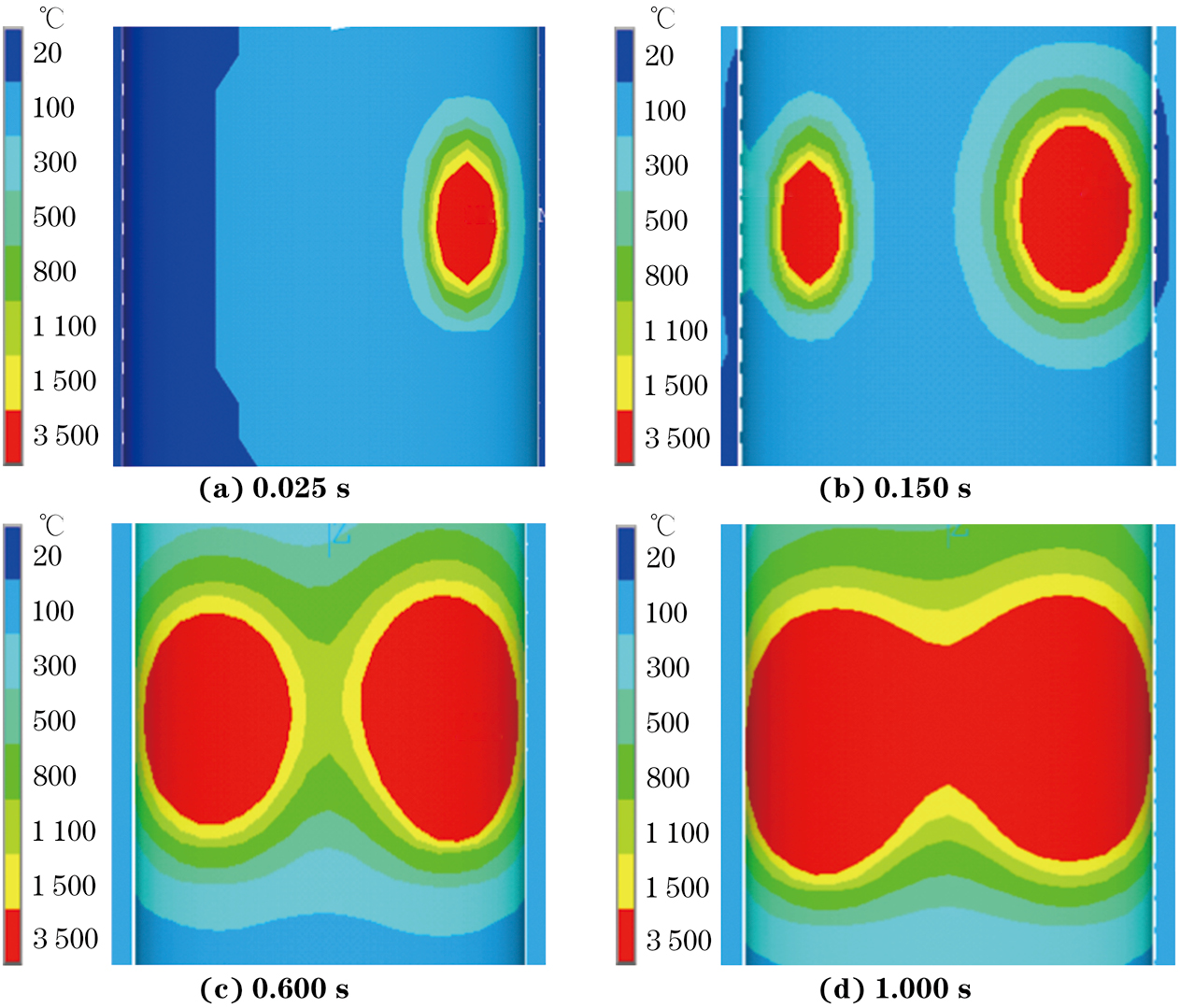
图 10 考虑侧壁热源时模拟得到不同时刻焊接接头的横截面温度场Figure 10. Simulated temperature fields on cross-section of welded joint at different times with considering sidewall heat source: (a) 2.750 0 s (arc located on left side of bead); (b) 2.812 5 s (arc located in middle of bead) and (c) 2.875 0 s (arc located on right side of bead)由图11和图12可见:不考虑侧壁热源时,电弧在右侧引燃,形成熔池,但此时焊件的温度较低,起弧点的热量迅速散开,因此熔池尺寸较小;而后电弧向左侧摇动,熔池尺寸变大,由于电弧摇动速度较快,且在左右两侧停留时间较长,因此两侧首先形成熔池,而焊道中间未形成熔池;随着时间的推移,焊件温度不断升高,左右两侧的熔池不断扩大,在1.0 s时横跨整个焊道。在温度场稳定前,考虑侧壁热源时熔池的形成规律与不考虑侧壁热源的基本一致;在两侧壁停留时,考虑侧壁热源时一部分电弧热量分解给了侧壁热源,因此接头上表面的熔池尺寸略小于不考虑侧壁热源时。
由图13和图14可以看出,考虑与不考虑侧壁热源2种条件下,温度场稳定后焊接接头上表面熔池形态变化不明显。这是因为焊接电流较大,熔池尺寸较大,而焊道较窄,电弧摇动频率高,且摇动范围有限,使得整个焊道受热均匀,因此熔池形态保持相对稳定。熔池两端凸出,造成这一现象的原因是,电弧在左右两侧壁停留时间较长,各达到60 ms且基本与从左侧摇动到右侧的时间一致。考虑与不考虑侧壁热源2种条件下的稳定熔池形态基本一致,说明在温度场稳定后是否考虑侧壁热源对焊接接头上表面的熔池尺寸影响不大。
4. 结论
(1)有限元模拟得到考虑侧壁热源时焊缝截面左右两侧壁熔合线最高点的深度与试验结果间的差值明显小于未考虑侧壁热源时,考虑与不考虑侧壁热源2种条件下,焊接接头不同测试点的热循环曲线的变化趋势与试验结果基本吻合,峰值温度的最大相对误差分别为1.8%和3.4%,可知考虑侧壁热源的热源模型能更加准确地描述摇动电弧窄间隙GMA焊过程中的热源分布特征。
(2)考虑侧壁热源时模拟得到底部熔深略小于未考虑侧壁热源时,而侧壁熔深则略大,但尺寸变化均小于0.1 mm;考虑侧壁热源主要影响两侧壁的熔合线最高点的位置。温度场稳定前,考虑侧壁热源时熔池的形成规律与不考虑侧壁热源的基本一致,但接头上表面的熔池尺寸略小于不考虑侧壁热源时;而温度场稳定后,是否考虑侧壁热源对焊接接头上表面的熔池尺寸影响不大。
-
图 9 未考虑侧壁热源时模拟得到不同时刻焊接接头的横截面温度场
Figure 9. Simulated temperature fields on cross-section of welded joint at different times without considering sidewall heat source: (a) 2.750 0 s (arc located on left side of bead); (b) 2.812 5 s (arc located in middle of bead) and (c) 2.875 0 s (arc located on right side of bead)
图 10 考虑侧壁热源时模拟得到不同时刻焊接接头的横截面温度场
Figure 10. Simulated temperature fields on cross-section of welded joint at different times with considering sidewall heat source: (a) 2.750 0 s (arc located on left side of bead); (b) 2.812 5 s (arc located in middle of bead) and (c) 2.875 0 s (arc located on right side of bead)
表 1 Q370qE钢的热物理性能参数
Table 1 Thermophysical performance parameters of Q370qE steel
温度/℃ 密度/(g·cm−3) 热导率/(W·m−1·℃−1) 比热容/(J·kg−1·℃−1) 20 7 800 51.9 450 100 7 740 51.1 499 300 7 700 46.1 565 550 7 610 37.5 705 750 7 550 30.6 1 080 1 000 7 490 29.4 437 1 200 7 400 29.7 400 -
[1] 林三宝,蔡笑宇,季相儒. 厚板窄间隙焊接技术研究进展[J]. 机械制造文摘(焊接分册),2017(5):33-38. LIN S B ,CAI X Y ,JI X R. Research status of narrow gap welding for thick plates[J]. Welding Digest of Machinery Manufacturing,2017(5):33-38.
[2] 赵博窄间隙MAG焊电弧行为研究哈尔滨哈尔滨工业大学2009赵博. 窄间隙MAG焊电弧行为研究[D]. 哈尔滨:哈尔滨工业大学,2009. ZHAO BResearch on arc behaviors of narrow-gap MAGHarbinHarbin Institute of Technology2009ZHAO B. Research on arc behaviors of narrow-gap MAG[D]. Harbin:Harbin Institute of Technology,2009.
[3] WANG J Y ,ZHU J ,FU P ,et al. A swing arc system for narrow gap GMA welding[J]. ISIJ International,2012,52(1):110-114. [4] XU G X ,LI L ,WANG J Y ,et al. Study of weld formation in swing arc narrow gap vertical GMA welding by numerical modeling and experiment[J]. The International Journal of Advanced Manufacturing Technology,2018,96(5):1905-1917. [5] WANG J Y ,JIANG Y Q ,ZHU J ,et al. Development of swing arc narrow gap GMAW process assisted by swaying wire[J]. Journal of Materials Processing Technology,2023,318:118004. [6] 黄健康,孙天亮,樊丁,等. TIG焊熔池表面流动行为的研究[J]. 机械工程学报,2016,52(18):31-37. HUANG J K ,SUN T L ,FAN D ,et al. Study on the surface flow behavior of TIG weld pool[J]. Journal of Mechanical Engineering,2016,52(18):31-37.
[7] GAO X S ,WU C S ,GOECKE S F ,et al. Numerical simulation of temperature field,fluid flow and weld bead formation in oscillating single mode laser-GMA hybrid welding[J]. Journal of Materials Processing Technology,2017,242:147-159. [8] LI W H ,YU R ,HUANG D C ,et al. Numerical simulation of multi-layer rotating arc narrow gap MAG welding for medium steel plate[J]. Journal of Manufacturing Processes,2019,45:460-471. [9] ELMESALAMY A S ,ABDOLVAND H ,WALSH J N ,et al. Measurement and modelling of the residual stresses in autogenous and narrow gap laser welded AISI grade 316L stainless steel plates[J]. International Journal of Pressure Vessels and Piping,2016,147:64-78. [10] 张华军,张广军,蔡春波,等. 摆动焊接动态过程温度场数值模拟[J]. 焊接学报,2008,29(2):69-72. ZHANG H J ,ZHANG G J ,CAI C B ,et al. Numerical simulation on temperature field of dynamic welding processing with weaving[J]. Transactions of the China Welding Institution,2008,29(2):69-72.
[11] 胥国祥,潘海潮,王加友. 摇动电弧窄间隙GMAW焊温度场数值分析模型[J]. 焊接学报,2017,38(10):55-60. XU G X ,PAN H C ,WANG J Y. Numerical analysis model of temperature field in swing-arc narrow gap GMAW[J]. Transactions of the China Welding Institution,2017,38(10):55-60.
[12] LIANG G D ,QIN G L ,CAO P Z ,et al. Numerical investigation of sidewall penetration in narrow gap oscillating laser welding process[J]. Optics & Laser Technology,2024,170:110282. [13] 张李祥中厚板窄间隙摆动激光填丝焊工艺参数优化与数值分析徐州中国矿业大学2023张李祥. 中厚板窄间隙摆动激光填丝焊工艺参数优化与数值分析[D]. 徐州:中国矿业大学,2023. ZHANG L XOptimization and numerical analysis of process parameters for narrow gap swing laser wire-filling welding of medium and heavy plateXuzhouChina University of Mining and Technology2023ZHANG L X. Optimization and numerical analysis of process parameters for narrow gap swing laser wire-filling welding of medium and heavy plate[D]. Xuzhou:China University of Mining and Technology,2023.



 下载:
下载: